Exhibit Contents
Part 2
The first reception of the Timaeus in the sciences and natural philosophy we find in Aristotle, especially in his Physics and in his De Caelo. For Aristotle the Timaeus was especially important, among other reasons, because, as Aristotle states in the Physics passage shown, Plato was the only one who had dealt with the problem of space/place in a scientific way before. A very special kind of reception is the text Peri psychas kosmô kai physios, which allegedly shows some of the pre- rather than of the afterlife of the Timaeus. Written in the first century AD it was passed off as a pre-Platonic text by the Pythagorean Timaeus of Locri, on which Plato’s Timaeus was supposed to have drawn. The Neoplatonists were the first to accept it as an authentic document; but others did as well, as can be seen from the fact that Henricus Stephanus’ edition has this text printed in between Plato’s Timaeus and Critias; this is the reason why the Stephanus pagination of the Critias is not immediately continuous with that of the Timaeus.
The Timaeus drew a strong reception also during the Middle Ages. One essential reason for this was its obvious compatibility in many respects with the book of Genesis. The Timaeus was paired up with Genesis probably already as early as the 2nd century BC by Aristobulus, an intertwining anchored by Philo of Alexandria who focused on the parallels between Platonic cosmogony and the account of creation in the first book of Moses13.
At times the differences between these two accounts of creation were stressed more than the similarities14, notably the difference between god as the single cause for the creation in Genesis and the three principles necessary for the creation in the Timaeus, the demiurge, the receptacle and the model (Forms). Especially Ambrose in his exegesis of Genesis in the 4th AD century pointed these differences out as “errors” of Plato. Ambrose thought these “errors” had already been refuted by Moses since Genesis starts out with “in the beginning god created heaven and earth”, thus, according to Ambrose, implying that everything, even the independent first principles of the Timaeus, is in fact created by god.
However, Ambrose’s student Saint Augustine15and later on also Boethius16 focused on the similarities again — Augustine explicitly mentions as a point of agreement the same beginning passage from Genesis used by Ambrosius to point out the incompatibility of the Timaeus and Genesis17 — and thus prepared the way for the Timaeus into the Middle Ages. The 12th century was especially crucial in the reception of Plato: the first half of the century marked the climax of his reception in the Middle Ages, as can be seen in particular with the School of Chartres where Bernard of Chartres’ and William of Conches’ Glosae super Platonem were produced. In the second half, however, the study of Plato diminished and became either insignificant (thus in the translation movement of the 12th century Plato’s oeuvre beyond the Timaeus did not raise much interest) or suspect (as can be seen in the trial of Abelard where the preoccupation with Plato was regarded as a possible seduction away from Christ).18 While Aristotle gained more and more influence with his newly translated works in natural philosophy as well as metaphysics and ethics in the later part of the 12th century in addition to his already translated logical works, Plato and his Timaeus were increasingly marginalised. Overcoming this marginalisation and rediscovering Plato and his Timaeus as an important philosophical document is an essential feature of the Renaissance. And it is this new evaluation of Plato’s Timaeus which provided us with the core items of this exhibition.
In the Renaissance, the Timaeus is again read as being very close to the conception of Genesis; so e.g. Ficino identified Plato’s demiurge with the Christian creator god and the Forms with god’s own mind19; in the Ficino edition of Plato from 1556 Plato (in Neoplatonic tradition) is even addressed as being divine: “Omnia Divini Platonis Opera”. And Kepler in his Harmonice Mundi calls the Timaeus “a kind of commentary on the first chapter of Genesis, or the first book of Moses, converting it to the Pythagorean philosophy”. Some 200 years later Schelling actually proposed that the Timaeus was not written by Plato but by a late Christian author.20
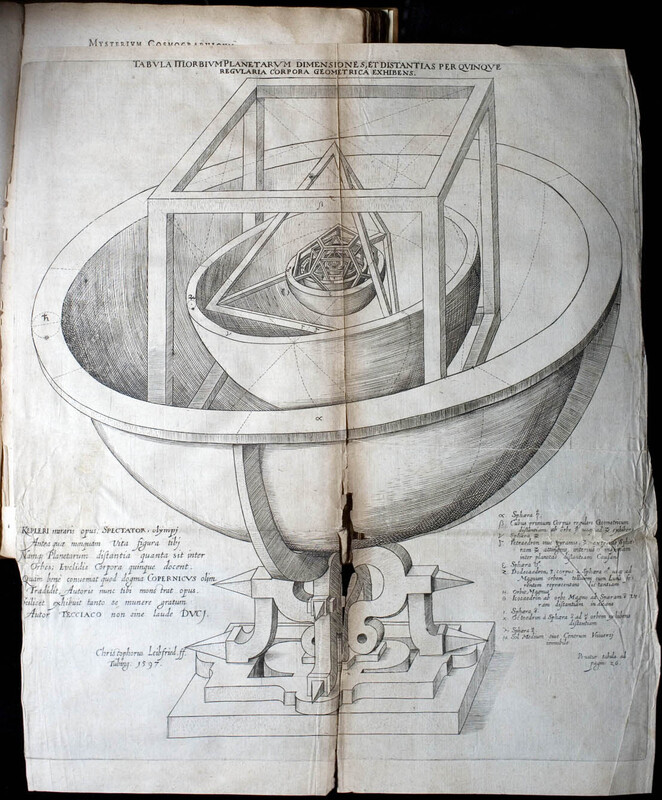
However, it is not only this new emphasis on its compatibility with Genesis that made the Timaeus a significant book for the Renaissance. It is also its combination of mathematics and physics that made it attractive for the newly reawakened sciences. Accordingly, Johannes Kepler uses the Timaeus to justify his own merging of mathematics and physics in his astronomy21which is a central point in his Astronomia nova. Nonetheless, Kepler did not simply take the Platonic text as an authority, as was done in the commentators’ tradition. Rather he considered the Timaeus as a resource to draw on for his cosmological questions. And the question which inspired Kepler to write his Mysterium Cosmographicum was how to give a rational account of the distances between the planets which one could attempt to calculate only once the sun was placed in the middle of the universe. The answer Kepler took from the Timaeus was the five Platonic solids and the relative distances between them which are achieved if they are nested in each other; we show the second edition which was expanded with notes by Kepler). The number of the solids (given Euclid’s proof in Elements book XIII, Prop. 18 that there can only be five such solids) explains why there must be exactly six planets (the five solids are needed to separate six spheres), and the geometry of their nesting gives us the distances – this enabled Kepler to retain his belief that god did everything for a cause, so also the arrangement of the universe, and to give a scientific model for the empirical data.
In this way, Kepler as one of the early defenders of the Copernican world view tried originally to give Plato a place in this new system which obviously contradicts Plato’s (and Ptolemy’s) geocentric view. One other aspect of Platonic astronomy Kepler had originally taken up is Plato’s insistence on uniform circular motion as being the one motion most akin to reason (Timaeus 34a) and hence the only one adequate for the motions of the heavenly bodies. Overcoming this Platonic idea by building on Tycho Brahe’s data led to Kepler’s famous first law that the planets revolve around the sun not in circular but in elliptical orbits having the sun as one focus (published in his Astronomia nova in 1609). The discovery of this first law helped to strengthen the heliocentric view of Copernicus who himself had followed Plato’s demand for uniform circular motion22for the heavenly bodies in an even stricter way than Ptolemy did centuries ago in his Almagest.
The edition of the Almagest on display is the version with which Copernicus was introduced to Ptolemy. It is a précis done by the Austrian astronomer Georg von Peuerbach and the German astronomer Johannes Müller von Königsberg (know as Regiomontanus). Included in Regiomontanus’ notes on the 12th book of the Almagest is his demonstration of an alternative to Ptolemy’s model of Mercury and Venus’ orbits relative to the sun; this demonstration is believed to have actually assisted Copernicus to postulate that the planets move around the sun.23
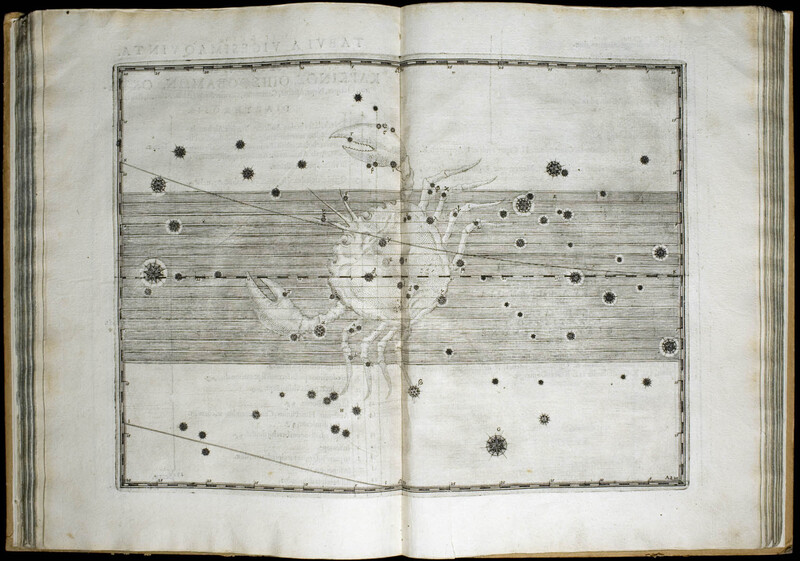
While Ptolemy could not strictly adhere to Plato’s fundamental claim of the uniformity of heavenly motion, he certainly observed Plato’s dictum that astronomers should try to find a reasonable hypothesis which would “save the phenomena”, salvare apparentia24 (i.e. a rational account should be given of all the phenomena that can be observed) not only for the motions of the planets25, but also for the stars. His placement of the stars within constellations seems to be based on star maps, or perhaps even on a celestial globe (the Farnese globe at Naples surviving from ancient times is generally believed to be a Roman copy of an earlier Greek one, cf. also the celestial globe in the exhibition. This tradition of star maps building on Ptolemy is taken up again in the Renaissance, the first printed one is made by Albrecht Dürer, numbering the stars according to Ptolemy’s list. The first person to produce what is considered the first star atlas was Johannes Bayer in his Uranometria from 1603. He extended the 48 constellations tabulated by Ptolemy by 12 new constellations of the Southern hemisphere found by the first Dutch expedition to the East Indies and improved the accuracy of the old constellations with the help of Tycho Brahe’s data.
Works Included
This volume contains an edition of Marsilio Ficino’s Platonis Opera Omnia that was emended and collated with a Greek text by Simon Grynaeus (1493-1541). Grynaeus was a renowned Protestant humanist who shared friendships with Erasmus and Budé. He is the first major revisor of Ficino’s work. Grynaeus’ edition of Ficino’s text was first published in 1532. In his preface, Grynaeus proclaims his admiration of Ficino and his desire to make Platonic doctrine more available to all. He justifies the new edition by stating that he had collated Ficino’s work with a Greek exemplar (the Aldine Greek edition). However, Grynaeus probably also disliked Ficino’s style; he actively attempts to revise Ficino’s Latin in accordance with classical usage. The volume shown here is a reprint of the 1532 edition with the addition of a new index, printed by Giovanni Maria Bonelli at Venice in 1556. The pages displayed translate the Timaeus passage 18a-24d– the beginning of Critias’ account of Solon’s visit to Egypt. A fine Italic hand has written topical captions in the margins.
This book is part of the editio princeps of the Aristotelian corpus, which Aldus Manutius (1449/1450-1515) published himself in five folio volumes at Venice in 1495. Manutius was famous for his devotion to ancient Greek literature; he wished to save the Greek corpus from further loss by committing as many works as possible to type. He also developed the typeface style known as italic. Manutius’ edition of Aristotle was the first major Greek prose corpus to be printed in its original language and set the standard for the development of Greek type in the fifteenth century. The pages displayed include a passage from De Caelo in which Aristotle mentions the receptacle described in Plato’s Timaeus as the receiver of all things. This passage, De Caelo 306b15-22, begins in line 10 on the left page shown with the words
Ἀλλ’ ἔοικεν ἡ φύσις.
This manuscript contains, among other material, anonymous commentaries on a number of Aristotelian works. Multiple fifteenth-century hands are represented in the manuscript, and some of the scribes give their names. Judging by the extensive notes and highlighting throughout the volume, this codex probably belonged to a student. The pages displayed contain a commentary on book IV of Aristotle’s Physics with many marginal notes. The right page includes a passage discussing Plato’s conception of space and matter (which Aristotle mentions in Physics 209b). While the cursive hand is very difficult to read, there is an abbreviation (plto) roughly in the middle of the right column that probably represents “Plato.” It is also possible to make out the phrase antiqui phī (“ancient philosopher,” the second word being an abbreviation for philosophi) in this section.
Published at Frankfurt by Erasmus Kempfer, this volume contains the second edition of the astronomical work, Mysterium Cosmographicum (originally published in 1596) by Johannes Kepler (1571-1630), along with copious notes by Kepler himself. The Mysterius Cosmographicum was the first published defense of the Copernican heliocentric system. It was in this work that Kepler believed he had uncovered God’s geometrical plan for the universe – he proposed a model of the solar system using nested Platonic solids. The page displayed illustrates his distinctive model of the universe.
This volume is a first edition of Johannes Kepler’s Harmonices Mundi, printed by Johannes Plancus at Linz (Austria) in 1619. In this work, Kepler explains his vision of harmony and congruence in geometrical forms and in the physical world. This vision of harmony focuses upon his conception of musica universalis – the “music of the spheres,” which had been studied by earlier astronomers (including Ptolemy). He also details his discovery of the Third Law of planetary motion. The pages displayed include a lengthy quotation from Proclus and a marginal note indicating that Kepler considered Plato’s Timaeus as a commentary on the biblical book of Genesis.
The Uranometria, published by the lawyer and amateur astronomer Johann Bayer (1572-1625), was the first astronomical work to be considered a collection of star maps (rather than symbolic pictures). It was printed at Augsburg by Christopher Mangus in 1603, and the elaborate copperplate engravings were done by Alexander Mair. One important feature of Bayer’s atlas is his new system of stellar nomenclature—he assigned Greek letters to brighter stars, generally by order of magnitude. The plate displayed is Bayer’s map of the constellation Cancer.
Georg von Peurbach (1426-1461) began work on this condensed version of Ptolemy’s Almagest; his pupil and friend Johannes Müller von Königsberg (1436-1476), known by the pseudonym Regiomontanus, finished it. Printed at Venice by Johannes Hamman in 1496, the book became a renowned astronomical guide. Columbus and Copernicus were introduced to Ptolemy’s work via this epitome. The work includes a woodcut portrait of Ptolemy and Regiomontanus underneath a large celestial sphere. This portrait shows Ptolemy reading from the Almagest while Regiomontanus listens and points to the celestial sphere – a representation of the heavenly order described in Ptolemy’s work. The pages displayed here are from the eighth book of the epitome, the section covering positions of the stars.